In the introductory solid state physics courses, lattice vibrations in a crystal are typically introduced by considering first a hypothetical one-dimensional chain of atoms, which can be solved fully making it easier to grasp the idea of phonons (quanta of lattice waves) without really worrying about the complexities of a real three-dimensional crystal.
The ditto idea was originally advanced to understand the behavior of magnets. Analogous to the one-dimensional chain of atoms, one can envisage a chain of magnetic dipoles; each dipole being a tiny atomic magnet. If there is just one unpaired electron on each atom of the chain, the resulting chain is called the spin-1/2 chain, one-half being the spin quantum number of the electron. The interaction between the neighboring spins of the chain is governed by the rules of quantum mechanics. If the sign of interaction is such that the spins prefer to align in the same direction they are said to be ferromagnetically coupled; if however they prefer anti-alignment their coupling is called antiferromagnetic. In metallic iron, for instance, the spins are coupled ferromagnetically; therefore, when cooled below a critical temperature, the ferromagnetic interaction between the iron spins overcomes the thermal randomness to form a long-range ordered state where all spins point nearly in the same direction. This alignment gets more and more perfect as the material is cooled to absolute zero temperature.
The investigations into spin chains started as early as in the year 1934. Ernst Ising, on the advice of his PhD supervisor Wilhelm Lenz, made the first attempt to find the ground state of a spin chain. To make things simpler he assumed that the spins at each site can either point up or down and allowed only nearest neighbors to interact. This model was later called the Ising model. Ising found that the spins of the chain show no long-range ordering at any finite temperature no matter how strongly the spins interact! This, of course, was quite disappointing for Ising because he expected to find a phase transition at a finite temperature, as happens in any real three-dimensional magnets. This counterintuitive result, however, gave birth to an interesting area of low-dimensional magnetism. This and the subsequent work made it clear that magnetism in spatial dimensions less than three, is not only unique but also full of surprises. The experimental realization of spin chains took almost three to four decades after the seminal work of Ising. Excellent magnetic one-dimensionality can be found in many organic and inorganic solids where spin-spin interactions are present only along specific crystal directions.
The real interest in quantum spin chains started with the work of Duncan Haldane of the Princeton University, who also shared the Nobel Prize in Physics last year for his theoretical work on spin chains. He conjectured that the antiferromagnetic spin chains with integral spins (i.e., spin = 1, 2, …) will have a gap in their excitation spectrum (i.e., the excited states are separated from the ground state by a finite energy gap), while those with a half-integral spin (spin = 1/2, 3/2, …) will be gapless. Since then this conjecture has been experimentally tested to be true on several spin chain systems.
Interestingly, the gapping in the integer spin chains is a consequence of a topological term in the spin Hamiltonian. This gap therefore is topologically protected against any kind of disorder or impurities present in the chain. On the other hand, the gapless behavior of the half-integer chains has no such topological protection. In particular, in the spin-1/2 chain, where the smallness of the spin-size brings in additional quantum effects through the quantum uncertainty principle, the ground state and the spin excitations are qualitatively reorganized in the presence of disorder or impurities.
In the year 2013, an international team of researchers, including Dr. Surjeet Singh from IISER Pune, started testing the gapless nature of the spin-1/2 chain in the presence of very tiny concentration of impurities. They found that as small as 1% of spin-1 impurities in the antiferromagnetic spin-1/2 open up a sizeable spin gap in the excitation spectrum. In a spin-1 chain, gapping is expected as conjectured by Haldane. But why merely 1% of spin-1 impurities in the spin-1/2 chain yields a gap raised several questions about our understanding of the spin-1/2 chains. It was argued that nickel impurities disrupt the translational invariance of the spin 1/2 chain leading to finite-size effects. The quasiparticles (called spinons) associated with the excitations in the spin-1/2 chain find themselves quantum confined between the impurity-spins, which results in the spin pseudogap; almost akin to what happens to a free-particle when it is confined in a box of finite-length.
In a recent article published in the journal Physical Review Letters, Dr. Singh, who lead the study along with his PhD student Koushik Karmakar, and a team of researchers from Paul Scherer Institute in Switzerland and from IFW Dresden, Germany, investigated the ground state and spin excitations of the antiferromagnetic spin-1/2 chain in the presence of a spin-1/2 impurity. In this paper, Karmakar et al. show that in the presence of a spin-1/2 impurity the spin excitation spectrum remains gapless. This is not surprising since the spin-1/2 impurity perturbs the spin-1/2 chain only weakly. This is because in the presence of impurity, the translational invariance remains and only the magnetic interaction strength in the neighborhood of the impurity spin changes marginally. The unexpected effect of the impurity, however, is that less than 1% of added impurities increase the weak antiferromagnetic ordering temperature of the chains significantly. Here one should note that, all the real spin chain systems do show 3D magnetic ordering due to finite interchain magnetic coupling. However, the ordering temperature is much lower as compared to magnetic interaction within the chains. This result is unexpected because no new exchange paths are created upon replacing the host spin-1/2 by an impurity spin-1/2. Therefore, the observed doubling of the ordering temperature is not merely an interchain effect; and cannot be accounted for on the basis of the quantum mean-field theory of weakly coupled chains.
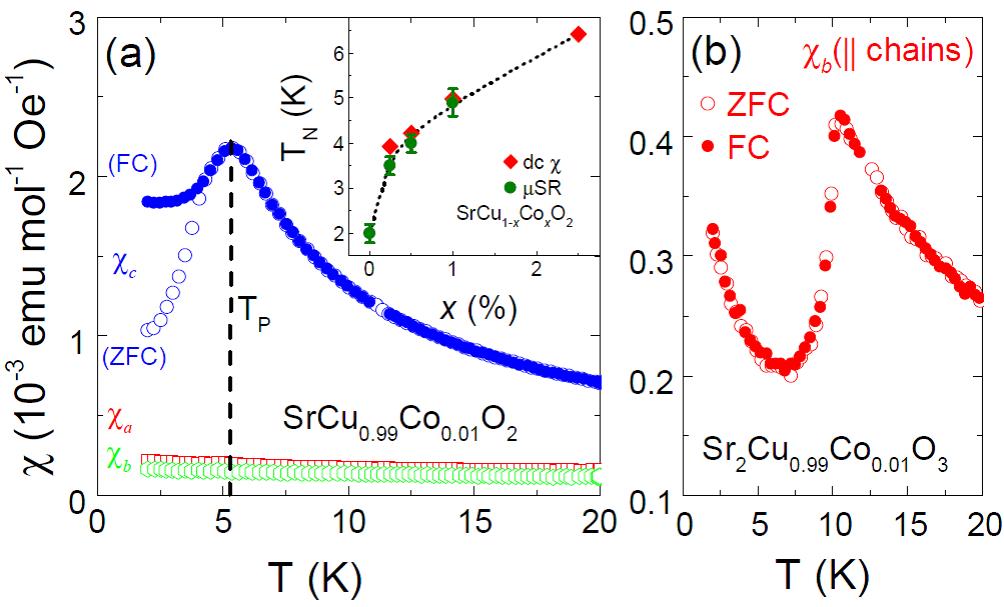 |
Magnetic susceptibility shown as a function of temperature (T). The spin chain systems examined are SrCuO2 (left panel) and Sr2CuO3 (right panel). In both systems, the Cu ions have a spin 1/2, and in the three-dimensional crystal framework they form well-isolated chains. The chains in SrCuO2 are zigzag shaped, while in Sr2CuO3 they are linear. Data shown here are for the 1% Co-doped crystals. The sharp peak in the susceptibility marks the Néel ordering temperature. In SrCu0.99Co0.01O2 the transition temperature near 5 K is up from a value less than 2 K in the pristine compound (see inset). Similar behavior is witnessed in Sr2Cu0.99Co0.01O3, where the transition temperature increases from 5.4 K in the pristine crystal (not shown) to near 11 K in the doped crystal. The open and filled circles represent two different measurement protocols: Field-Cooled (FC) where the crystal is cooled to the lowest measurement temperature under an applied magnetic field; and Zero-Field-Cooled (ZFC) where the cooling is done under a nominally zero-field before starting the measurements. In the left panel, the ZFF-FC branches split to reveal the presence of a weak geometrical frustration in the zigzag chains. In the right panel they overlap in line with the fact that in a linear chain there is no geometrical frustration. |
Scientific details: Karmakar et al. measured bulk magnetization at low-temperature using a Physical Property Measurements Systems at IISER Pune on single crystals of spin chain materials grown in-house using the traveling-solvent floating-zone technique. To study the excitation spectrum, inelastic neutron scattering experiments were performed at the Paul Scherrer Institute (PSI), Switzerland, under the Indo-Swiss personnel exchange program in collaboration with the group of Prof. Christian Rüegg. To corroborate the long-range ordering elastic neutron scattering experiments and muon-spectroscopy experiments were also carried out at PSI (lead by Dr. Giacomo Prondo). Their experiments revealed that the impurity spin has a strong preferential orientation parallel to the spin chain as opposed to the behavior of the host spin which shows no such preference. They found that the orientational preference of the impurity spin is transmitted to the whole chain. What is remarkable is that merely 0.25% of impurity concentration effectively switches the symmetry properties of the whole chain from Heisenberg to Ising-like. Since examples of Ising-like chain, as opposed to Heisenberg or Ising chains are rare, this research shows how by adding a tiny bit of Ising impurities to the Heisenberg chain one can tune the chain to behave as Ising-like (i.e., in between these extreme limits). The paper also shows how these enhanced correlations in the presence of impurity can be successfully exploited to unveil the weak geometrical frustration in the zigzag shaped spin-1/2 chains. The central result of the paper is summarized in the figure. Caption to the figure provides the specific details of the experiment.
The paper is accepted for publication in Physical Review Letters (PRL) [Karmakar et al. Phys. Rev. Lett. 118, 107201 (2017)].
-By Dr Surjeet Singh, IISER Pune





